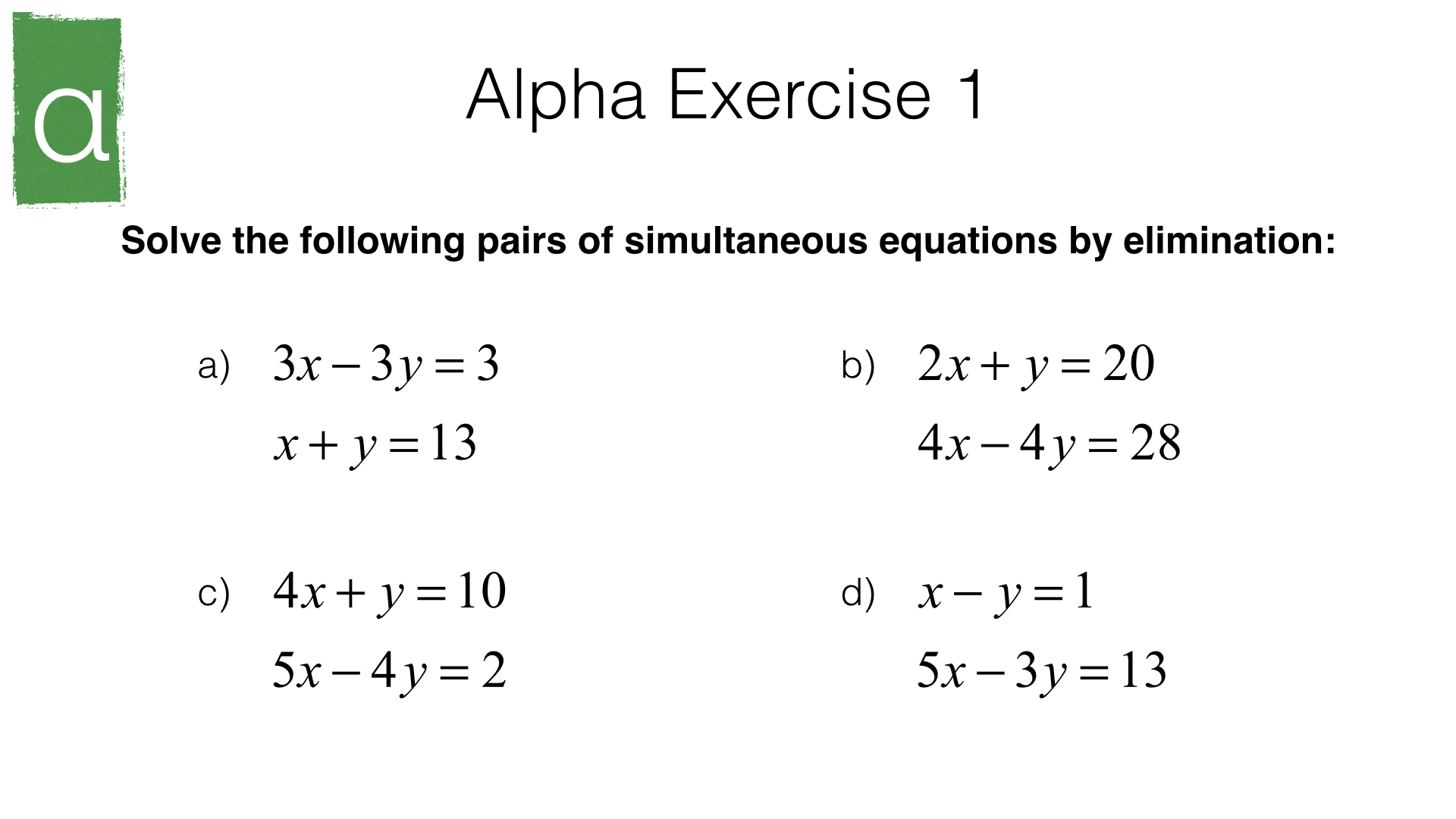
Get your free simultaneous equations worksheet of 20+ questions and answers. A quadratic equation contains terms that are raised to a power that is no higher than two. Each of these equations on their own could have infinite possible solutions. Look out for the simultaneous equations worksheets and exam questions at the end. To verify the point (4, -1), substitute the same in the equations. Multiplying the equation 2 by 2, to make the coefficient equal in both equations.

Methods for Solving Simultaneous Equations

• Let us now understand how to solve simultaneous equations through the above-mentioned methods.
• From equation (1) and equation (2) we will determine the value of x and y.
• We need to convert this worded example into mathematical language.

In above set first equation comes with second degree so this set will be called nonlinear simultaneous equations. The aim of this section is to understand what are simultaneous equations and how we can solve them? After reading this section we will be able to write down a word problem in the form of simultaneous equations and be able to find out the solution. When you have plotted the graphs, you can work out the values of \(x\) and \(y\) that solve the equations.

Make the coefficients of one of the variables the same in both equations

While it involves several steps, the substitution method for solving simultaneous equations requires only basic algebra skills. Simultaneous equations or a system of equations consisting of two or more equations of two or more variables that are simultaneously true. Thus, for solving simultaneous equations we need to find solutions that are common to all of the given equations. Amongst the various types, the article will focus on simultaneous linear equations. Linear equations in one variable and multiples are equations of degree 1, in which the highest power of a variable is one. In this article, we are going to learn different methods of solving simultaneous linear equations with steps and many solved examples in detail.

Worked example 10: Simultaneous equations

A pair of linear equations can also be solved using the graphical method. The graph of linear equations in two variables represents straight lines in the two-dimensional cartesian plane. The intersection point of these lines gives us the common solutions to our simultaneous equations. Let us understand how to solve simultaneous equations graphically.

Go through the solved example given below to understand the method of solving simultaneous equations by the elimination method along with steps. Here, you will learn the methods of solving simultaneous linear equations along with examples. Many times, we find the solution but forget to check that even it is true or false.

Simultaneous Linear Equations

We now have two equations of straight line graphs, which we can plot. Neither the \(x\) nor the \(y\) will be eliminated by adding or subtracting these equations as they stand. By adding the two equations together we can eliminate the variable y. For each of the simultaneous equations examples below we have included a graphical representation. When we draw the graphs of these two equations, we can see that they intersect at (1,5).

Now, let us discuss all these three methods in detail with examples. According to method first make the coefficient same of a one variable, here we make the same coefficient of x. See both the equations are true at a same time for single value of x and y. Notice we now have equations where we do not have equal coefficients (see example 3). In this example this is the letter \(y\), which has a coefficient of 1 in each equation. Consider the first equation as a reference (we can take any one of the equations).

In this method, we first try to represent both variables in terms of another variable arriving at equations in one variable which is trivial to solve. See the examples below for how to solve the simultaneous linear equations employment expenses of transport employees using the three most common forms of simultaneous equations. Below is the solved example with steps to understand the solution of simultaneous linear equations using the substitution method in a better way.

But you can use two equations together, if they have the same two unknowns, to make one equation that has only one solution. Subtracting the second equation from the first equation leads to a single variable equation, which determines the value of y . Substitute this value into either equation to determine the value of x . So our first step in eliminating one of the variables is to make either coefficients of h or i the same. 5Check your answer by substituting both values into either of the original equations.